Mathematics, often perceived as too abstract and detached from everyday life, is a nightmare for a growing number of students who, subsequently, reject it. According to Michel Broué, former director of the Institut Henri-Poincaré, “school has turned maths into a science for fools, made up of rote learning of techniques and application of abstract rules without knowing why”, whereas this discipline, again according to Michel Broué, is “the very place of imagination, intuition and aesthetics” (Le Point Hors-Série, 2017).
This dislike of mathematics is far from irreversible. Nevertheless, in order to reverse this disposition, it seems necessary to adopt new teaching methods and devices that no longer lead to discouragement, in order to make mathematics a tool for understanding and apprehending the world around us better.
To face this challenge, educators have developed several methods, the main ones being:
3.1 – Mathematical methods :
3.1.1 – The Singapore method
When the city-state of Singapore gained independence in 1965, it equipped its schools with textbooks from Western countries that covered different mathematical researches and pedagogies (such as, those of Bruner, Polya and Montessori). Making mathematics and science learning a national priority in the early 1980s, teachers were commissioned to produce a synthesis of the most effective methods. Over the next fifteen years, the developed method continued to be tested and adjusted. It is now an integral part of the country’s education system from kindergarten to primary school.
Indeed, the starting point of this method is that one should not learn mathematics but, above all, understand it. In order to memorise it, it is necessary to understand the meaning and usefulness of the formulae first. Learning them by heart is, in a way, counterproductive. It certainly enables us to apply them mechanically at first, but inhibits us from remembering and applying them again at a later stage.
The method is progressive in the sense that it starts with simple concepts that can be illustrated with pictures. As one progresses, the concepts become more complex and abstract. Learners must be able to construct and explain logical reasoning on their own.
The Singapore Method is based on three fundamental axes:
- Modelling, which consists of getting the learner to construct a plan, a representation of the problem.
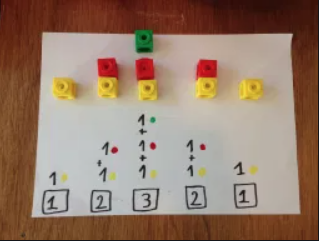
- The “concrete-imaged-abstract” approach, which consists of using everyday situations, cubes or other objects to be manipulated and then representing them using circles, bars or dots and finally writing them down using numbers and symbols.
- Verbalization allows the student to explain how they will solve the problem by describing each step of the reasoning
3.1.2 – The Assimil method
This method was created in 1929 by a French language teaching publisher. It is essentially based on intuitive assimilation and thirty minutes of daily work.
The principle is simple: wherever the students are, they can read or listen to formulas and theorems, which allows him to become familiar with the language of mathematics. After each lesson, the students have to practice for thirty minutes. Less than that would be ineffective but more than that would seem like a chore.
Each Assimil book contains between 60 and 150 lessons. Every 5 to 6 chapters an explanatory lesson is proposed in order to repeat the essential points studied in the previous lessons.

3.1.3 – The Berlitz Method
The aim of this method, created in 1878, is to enable the learner to think concretely about mathematics and to love it. Learning can take place in two different ways:
- Individually, so that the learner has the choice of the volume and time of his mathematics course(s) during the week.
- In groups of three learners of the same level who have the same objectives, which can limit some biases such as inferiority complexes that can lead to blockages.
Courses, whether individual or group, must take place in a Berlitz-affiliated institution.

3.1.4 – The Kumon Method
This method was developed by the Japanese mathematician Toru Kumon in 1954 in order to improve his son’s performance in mathematics at his wife’s request.
The basic principle is that everyone has an untapped potential that can be revealed. This is achieved by increasing the learner’s confidence in themselves and their skills through self-learning.
The method proceeds in stages, i.e., each learner integrates a notion, one after the other. To validate or not the learning of a notion, the learner takes a test where their speed of execution is examined.
The principle underlying this method is to respect the learning pace of each child by placing them in front of a concrete example, so that they can form a mental image that can be generalized and applied to other operations
3.2 – Digitized methodologies to teach Mathematics in Kindergarten
In order to bring mathematics to life, the introduction of a stimulating teaching method that establishes the link with the surrounding world has been deemed necessary. We have seen that other forms of didactics have been developed to meet this objective. However, the emergence of new technologies, and their popularization, have transformed our social practices and our understanding of the world. Moreover, it cannot be overlooked that these new technologies have also had a direct impact on the evolution of mathematical sciences and their practice.
This transformation, although rapid, has been through a lot of stages. Indeed, the enrichment of mathematics learning was initially only envisaged through calculators and professional software such as Excel. “In basic schooling, it is mainly calculators, spreadsheets and dynamic geometry software, as well as microworlds such as Logo” (Artigue, 2011). It cannot be denied that the contribution of these technologies has largely contributed to developing “the possibilities of experimentation, visualisation and simulation; they have modified the relationship to calculation and to geometric figures. They have brought school mathematics closer to the outside world by making it possible to deal with more complex data and more realistic problems” (Artigue, 2011). Nevertheless, it is unfortunate that this contribution has remained particularly underrepresented in basic schooling, and more particularly in kindergarten, due to the lack of training in these technologies for teachers.
More recently, the emergence of the internet and mobile technologies has opened up a new field of research for mathematics learning, both for students and teachers (distance education and training, free access to a wide variety of resources, creation of communities of learners and teachers among other new possibilities). “It is particularly important to take advantage of these possibilities for mathematics education, especially since it seems that their integration does not pose the same difficulties as the above-mentioned technologies, as they do not affect practices in a similar way” (NMC Horizon Report, 2017).
There needs to be an investment in the field of digital technologies in order to develop tools and even methods that would facilitate the learning of mathematics from kindergarten. “Quality mathematics education for all cannot be achieved without the production of quality resources: resources for students and resources for teachers”.
Technology in the classroom has come a long way. It is now more accessible than ever to create interactive lessons, implement participatory learning projects, provide personalised learning and organise classroom activities.
To date, there is a great number of methods and tools available in this regard, some of which are presented in the following non-exhaustive list:
3.2.1 – Virtual exhibitions and animations
Today, many museums such as the Palais de la Découverte in Paris, the Maison des mathématiques et de l’informatique in Lyon or the Maison des mathématiques in Belgium offer virtual exhibitions and animations that present mathematics in a fun and interactive way. However, very few are designed for kindergarten learners or even 6/7-year-old children. Nevertheless, they can be a support for teachers, a source of inspiration to create adapted workshops for their students. In addition, some institutions also offer online resources for teachers (files, teaching sheets or activities that can often be downloaded).
3.2.2 – Smartboards
Interactive and connected whiteboards can replace whiteboards in most classrooms. These boards offer students a much more enriching, fun and interactive experience. They can be used to display videos, photos, drawings and handwritten notes, and thus allow students to approach mathematics from different angles.
3.2.3 – Online games and apps
A recent study published in the journal Pediatrics by Shayl Griffith and colleagues found that the use of online games and apps was linked to higher mathematical ability in early childhood. However, it is important to remember that the teacher needs to think beforehand about how the game or application fits into the curriculum. According to Theresa Wills, an assistant professor of mathematics education at George Mason University in Fairfax, “It’s a great data point to see where kids are at and then teachers can use it to develop appropriate interventions for students who need more support”.
3.2.4 – Badging and gamification

Badging and gamification are motivational tools that reward the learner through the use of digital badges. After reaching a certain level of difficulty, the learner is awarded with digital badges. Thus, the acquisition of new badges forms a collection and transforms the learning process into a gamified process that is supposed to generate enthusiasm in the learner and encourage them to continue their efforts. However, for this method to be effective, it is necessary that the game/learning is linked to a specific pedagogical objective. Furthermore, while this method can create a form of emulation between learners, it can also prevent their cooperation and collaboration by creating rivalries.
The different technologies that can be used can all help to identify areas where learners are having difficulties and allow the lesson, or the pace of the lesson, to be adapted accordingly. In addition, they encourage interactivity and can therefore reduce the passivity of some learners. Furthermore, we all live in a digital and technological world. It is therefore essential to have access to and manipulate these tools from an early age “to generate a deeper understanding of the digital environment, allowing intuitive adaptation to new contexts and co-creation of content with others”.
Despite all the benefits that digital methods can bring, it must be admitted that they can also be a source of disadvantages. To be more specific, the first con is undoubtedly the fact that there is a certain inequality of access to digital technologies due to differences in infrastructure and equipment between different territories and between individuals. Many learners, whether in the classroom or at home, still do not have the necessary equipment to access digital content. Furthermore, and we will come back to this in more detail in our third part, not all teachers are trained and therefore do not master these technologies. Thus, the contribution of digital technologies to learning is, in these cases, non-existent.
It should also be noted that digital technologies may distract some learners, as their attention may be captured by other features available with these technologies. It is therefore imperative to counter this effect by making the course particularly lively and interactive. There are also concerns that these technologies are an obstacle to the development of verbal communication. Again, it is essential that these technologies are seen as tools, not as ends in themselves, promoting dynamism and interactions between learners and between learner and teacher. The keystone of school learning is, and will remain, the relationship between teacher and student.
Finally, as Rémi Brissiaud, a researcher at the Laboratoire Paragraphe, points out, “we should be careful about the race to develop digital resources. This rush risks being counterproductive because a question arises: is it reasonable today to mobilise so much goodwill, energy and funding to develop digital resources when, without this essential resource of reference to a common vocabulary to describe children’s digital progress, serious didactic errors are likely to be made?” (Brissiaud, 2014).
3.3 – Employing digitized tools and technological innovation for the creation of educational material by the kindergarten educators with the aim to reinforce mathematical learning in kindergartens
The use of digital technology is becoming more and more important in our daily lives and its integration into teaching practices is evident. The various technological innovations have led to pedagogical innovations by rethinking the way of teaching, i.e., the space given to interactions, autonomy and collaboration. Digitalisation should be seen as a tool that teachers can use to create their own teaching materials. It is not (or should not be) a substitute for the role of the teacher and their relationship with the learner.
The following is a list of different digital tools and innovations that can be used as complementary materials to support mathematics learning:
3.3.1 – Course platforms
The courses offered on the Khan Academy platform (https://www.khanacademy.org/math) consist of videos that illustrate the explanation of a concept or the solution of a problem, i.e., animations illustrate what is presented orally. The learner can then practise through a series of application exercises before taking the test that will allow to access the next course. The platform offers support for teachers as well. More precisely, it provides a monitoring tool that allows them to access the students’ achievements, the videos they have watched or to see the time they have spent on the platform. It should be noted that the content made available on this platform is completely free and without advertisements (the platform is financed by donations).

3.3.2 -Educational video platforms
The Lumni platform, provided by the French public broadcasting system (i.e., free of charge and without advertisements), makes available a number of files, including one on the fundamentals of mathematics (https://www.lumni.fr/dossier/les-fondamentaux-de-mathematiques). The latter is divided into several sections (geometry, numbers and calculation, units of measurement, etc.) which deal with the various concepts to be acquired through short videos. This platform enables learners to consolidate the elements studied in class and teachers to have access to expert resources.
Another example is the Canopé network, whose mission is to strengthen the action of the educational community in favour of student success. Through several platforms, the network offers resources for teachers. The “Les fondamentaux” platform (https://lesfondamentaux.reseau-canope.fr/discipline/mathematiques) in particular publishes a series of short, entertaining videos that can enrich a lesson by visually illustrating it.

3.3.3 -Edutainment sites
The Mathador site (https://www.mathador.fr), which is published by the Canopé network, offers two digital versions of its mental arithmetic game, which is designed for children aged 7 and above and uses multi-sided dice (10, 20, 6, etc.). “Solo” takes the form of a 30-level course in which the mental arithmetic operations become more complex as they progress. “Chrono” allows players to play alone or in pairs on the same screen as well as in a network against friends or random players. The player has 3 minutes to solve as many operations as possible, the more difficult the calculations, the more points they will get. Schools can take advantage of a “Mathador Classe” subscription that gives each pupil access to the two games, allows the class to take part in the Mathador competition (one test per week), and gives the teacher an interface where they can monitor the progress of their pupils.
The Matheros platform (https://matheros.fr/) is aimed at children from the age of 6 and also focuses on mental arithmetic. However, it is designed to be used with the whole class, as the teacher selects the exercises that the pupils should work on, either at home or in class. Each student plays a superhero whose goal is to liberate a city. The superhero will acquire new powers, i.e., new skills, depending on the student’s progress in mental arithmetic. The skill belts are obtained by going through four different stages: learning, practising, playing and finally validating the knowledge. This platform allows the teacher to follow the progress of each student and to adapt the following exercises according to their results.

The Number Race (http://thenumberrace.com/nr/home.php) is a free downloadable game for children aged 4 and above that teaches the basic concepts of numbers and arithmetic. This game is designed specifically for children with dyscalculia and reinforces the different mental imprints of the number (digit, word, quantity, position) and therefore its meaning.
The software is licensed under the Gcompris free license (https://gcompris.net/index-fr.html) and is aimed at children from the age of two. It is translated into 57 languages. Many edutainment activities related to mathematics are available. A teacher’s interface also allows the selection of games adapted to the level of the pupils or the material available.
Math Mathews is an application that helps someone learn multiplication tables. It follows the adventures of a brave pirate who, under a curse, is transformed into an octopus. In order to return Math Mathews to his original form, the child has to solve multiplications within a given time frame. As they progress, they will earn badges for each of the tables from 1 to 9.

3.3.4 – Innovative applications
Dragonbox Numbers and Big Numbers (https://dragonbox.com/products) are based on the Cuisenaire short rulers’ method, which represents the numbers from 1 to 10 in the form of bars. In these two applications, the short rulers have become Noums, a kind of friendly little monster that allows the learner to be introduced to numbers as a relationship between quantities. The learner can see how the Noum is made up (how many units), cut it up and thus give two smaller Noums. They can also make the Noums eat up each other to make a larger Noum. The three basic actions of problem solving (putting together, breaking down, and comparing) are thus earned here in a playful way and the association of gestures and concepts allows the cognitive processes to take root more effectively, thanks to the sensory dimension.

Edoki develops applications designed by Montessori teachers (https://montessori.edokiacademy.com/fr/catalogue/maths/) and are available in 8 different languages. The math-themed applications aim to reinforce counting, to teach how to write numbers and memorise their names, understand the concept of zero, and to introduce the first operations. Edoki has also developed a broader application, “La Maternelle Montessori”, which is accessible via a subscription and includes a myriad of educational mini-games.
3.3.5 – Applications involving the manipulation of solid objects
Marbotic (https://www.marbotic.com/smart-numbers/) has developed a method of learning numbers for children between 3 and 6 years old inspired by the Montessori method, which advocates the manipulation of physical objects to promote the acquisition of abstract concepts. Here, we can find coloured wooden pieces that can be interacted with on an application. Children enhance their motor skills while discovering numbers through their different representations (sound, image, calculation, etc.).
Osmo (https://www.playosmo.com/fr/shopping/games/numbers/) has developed a base and a sensor that attaches to the mobile device and detects the game pieces in front of it. The learner’s manipulations of the pieces are transposed onto the device’s screen, which helps to improve concentration and fine motor skills in addition to cognitive abilities.


3.3.6 – 3D modelling
3D modelling is of increasing interest to schools because it encourages transdisciplinary. It allows the initiation of projects that combine mathematics, technology and the plastic arts.


3.3.7 – Augmented reality
Augmented reality is a process that makes it possible to add virtual content to the real world, which can be viewed using a digital device. The advantage of augmented reality in learning is that learners, immersed in a dynamic environment, discover concepts in action and make them their own. The Merge Cube (https://mergeedu.com/cube) is one of these new solutions and is particularly accessible to young children. Through the “Frame” application, children have access to different written or illustrated mathematical challenges that they have to solve with the Merge Cube. Once they have solved all the challenges on one side of the cube, the cube is colored and the next challenges on the other sides must be completed.
3.3.8 – Books


Books have always been an excellent way of teaching complex concepts to young children, such as those that mathematics deal with. Some publishers, driven by the need to make mathematical concepts ever more comprehensible, have come up with original initiatives that we can separate according to three different approaches.
Firstly, we observe a narrative set up around mathematical objects allowing them to be better understood. This is the case with Petit cube chez les tout ronds, published by Mijade, in which the heroes are geometric objects with mathematical characteristics; or Raconte à ta façon, published by Flammarion, in which the characters of famous tales are represented by different geometric shapes that the children manipulate to reinvent the story.

The second category of books features children confronted with mathematical problems in everyday situations, for example in the collection Petites histoires Mathématiques published by Circonflexe. Solving these problems allows children to approach the first concepts of mathematics in a playful way, but also to develop their mathematical thinking.

Finally, the third category includes books that offer the opportunity to students to discover mathematics through the prism of the history of science and its illustrious characters. The aim of these stories is to provide role models in the field of sciences and render some concepts more accessible by demystifying these figures.
3.3.9 – E-books (or electronic books)
The release of the iPad by Apple in 2011, and then tablets in general, has changed the age at which electronic materials are accessed. With electronic screens now approaching the format of a book, educational content publishers have seized the potential of such devices and have begun to adapt their works for digital libraries.
Of all the varieties of ebooks, the EPUB fixed layout is the one most frequently chosen by publishers because of its cost of implementation. In fact, they are most often the adaptation of paper books, i.e., no additional work is done on the layout or on adding interactive or edutainment content. They are therefore the most economical and simplest solution for publishers specialising in illustrated books or books with complex layouts such as school textbooks.
However, new players have appeared on the market and are trying to develop these practices through specific work on the layout and the integration of interactive content in school textbooks. Among those who are taking this approach are Nathan – Mon cahier Maternelle, which offers a set of interactive and audio activities to introduce children to numbering and spatial orientation, with a reward system for each success; and Baobab Education in Canada, which has invested in the creation and implementation of a collection dedicated to mathematics for grades 3, 4 and 5. The books have been praised by teachers and awarded for their innovation and interactivity for the explanation of mathematical concepts.
3.3.10 – Audiobooks
Lunii, a new publisher that has developed its own material support, “Ma Fabrique à Histoires” for its audio and interactive books, has also recently taken an interest in educational and scientific content.
In the series of books “I calculate Step by Step with Kim and Tom“, everyday situations are presented. At the end of each chapter, a mathematical question is asked and, to continue the story, the children must choose a correct answer.
In the series of books “I calculate Step by Step with Kim and Tom“,everyday situations are presented. At the end of each chapter, a mathematical question is asked and, to continue the story, the children must choose a correct answer.

3.4 – Using technological innovation for the training of kindergarten educators
Technological developments and their integration into the field of education require teachers to learn new skills. Indeed, how can knowledge be transmitted with the help of tools whose operation is not mastered? Moreover, the issue of digital use reflects disparate situations both in terms of the skills of different teachers and in terms of the resources allocated for training and equipment. So how can we systematise the use of digital technologies in teaching methods, but also how can we harmonise practices so that their integration, or not, in education does not become an additional source of social inequality? This is a challenge that initial and in-service teacher training must now meet.
A recent study shows that two out of five education professionals have low or very low skills in new technologies. “Education today and in the future depends on the development of work communities in which diverse competences complement each other and the skills, attitudes, and knowledge of teaching professionals can be enhanced by professional development. Increasing the use of digital technologies in pedagogical practices should provide teaching professionals with recurring opportunities to develop their skills” (Hamalainen et al., 2020). Institutions, therefore, have a central role in offering more training courses that would enable teachers to acquire real techno-pedagogical expertise.
Tools for better teacher training already exist. Some of them may serve as a basis for thinking about new ones, more in line with the needs expressed by kindergarten teachers:
3.4.1 – M@gistère digital platform
Several training courses relating to the integration of digital technologies into learning are available: “Digital games for learning”, “Supporting pupils’ work with digital technology”, “Using the NIC in teaching”, “Teaching with digital technology: the digital kit”, “MOOC Tablet 2”, and “Digital technology and updated curricula – primary level”. As regards learning mathematics in kindergarten, the available material is not as extensive. To be more specific, only three courses are offered: “Giving meaning to ‘counting’ in kindergarten”, “The Construction of Numbers in the Middle and Upper Kindergarten” and “1,2,3… Building numbers”. It should be noted that no training program directly links the teaching of mathematics in kindergarten with digital technologies.
The principle of e-learning may at first glance be attractive, as it allows the teacher to organise their lesson as they wish. However, many see it as an additional burden, a tedious task to be carried out outside of one’s actual working time. Furthermore, as far as the M@gistère platform is concerned, the ergonomics are often questioned and do not facilitate immersion in the training. Moreover, there is little room for sharing and exchange.

3.4.2 – Enhanced e-books
Many textbooks in the form of digital books are available for teachers. They are most often adaptations of the same content in paper form. The Canopé network offers a wide range of books for kindergarten teachers on its website, including the very comprehensive “Enseigner les mathématiques en maternelle” (https://www.reseau-canope.fr/notice/enseigner-les-mathematiques-en-maternelle.html).

3.4.3 – The Mathematics laboratories
Although the “labomaths” were not initially intended to be implemented for kindergarten teachers, the approach seems interesting enough to be mentioned.
The “labomaths” have been developed to contribute to the professional development of teachers in teams. They are a place for ongoing training and reflection on the subject matter, didactics and pedagogy. They encourage interaction between teachers and allow the presentation of innovative practices and collaborative problem solving. The “labomaths” aim to reach out to their territory and establish relationships with partner establishments. They are also a place for producing resources (teaching resources, articles with disciplinary, didactic or pedagogical content, production of videos, sharing of content produced during events or sharing of experiences in various multimedia forms) which can be shared.


The use of new technologies most often requires technical skills and knowledge. However, there are digital solutions that provide teachers with both a tool and a method, i.e., how to implement the integration of this tool into the learning programme. This is the case with Marbotic and Dragonbox, both of which, as we saw earlier, are inspired by the Montessori method. Both offer teachers a pedagogical booklet that explains how the activities that can be carried out with their tools fit into the learning programme established for the class and guides them through this integration. For example, Marbotic, for “10 fingers”, explains in a preamble what skills are targeted in the kindergarten curriculum and how its application meets this requirement. A programme is then proposed in which, for each session, the content of the session, what the pupil learns and the conditions required for the proper conduct of the session are indicated. Dragonbox has a specific access for teachers on its website (https://dragonbox.com/educators) and provides various downloadable teaching guides for its games.